This is the second in my series of posts concerning lower bound for incidence problems (see the first post here). This post describes Elekes’ point-line construction, which is quite different from Erdős’ construction, although it yields asymptotically the same number of incidences. While preparing this post, I was quite surprised to discover that this construction yields constants better than those that are stated as the best known ones (e.g., in the research problems book).
Update 1 (11/26/2014): Frank the Zeeuw pointed out that this bound was already noticed in Section 1.1 of Roel Apfelbaum’s Ph.D. dissertation.
Update 2 (01/01/2018): It was pointed in this paper by Balko, Cibulka, and Valtr that the analysis of Pach and Tóth leads to the much better constant 1.27. This stronger bound is not stated in that paper due to a miscalculation.
Elekes’ construction is simpler and more elementary, in the sense that it only requires basic counting techniques (unlike Erdős’ construction which also relies on some number theory). As we shall see in the following posts, it is also easier to generalize to other types of planar curves. Moreover, unless I have some mistake, it seems to lead to better constants. Specifically, given 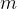 points and
points and 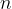 lines in the plane, the maximum number of incidences, as shown by Pach and Tóth, is at least
lines in the plane, the maximum number of incidences, as shown by Pach and Tóth, is at least  . In various places (such as the aforementioned open problems book) this is stated as the best known lower bound. The best known upper bound, obtained by combining a technique from the same paper of Pach and Tóth with a recent improvement by Ackerman, is about
. In various places (such as the aforementioned open problems book) this is stated as the best known lower bound. The best known upper bound, obtained by combining a technique from the same paper of Pach and Tóth with a recent improvement by Ackerman, is about 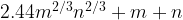 . Elekes’ construction leads to the improved lower bound
. Elekes’ construction leads to the improved lower bound  .
.
In both constructions the point set is a subset of the integer lattice. However, Erdős’ construction is based on a square lattice (i.e., of size 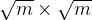 ), while Elekes’ contruction is a rather uneven lattice (except for the extreme case when
), while Elekes’ contruction is a rather uneven lattice (except for the extreme case when 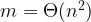 ).
).
To simply the explanation, we set  and
and 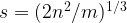 . We define the point set to be
. We define the point set to be
We define the line set to be
Notice that we indeed have
and similarly
Consider a line  that is defined by the equation
that is defined by the equation 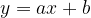 , for some constants
, for some constants  . Notice that for any value of
. Notice that for any value of 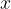 in
in 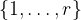 there exists a corresponding value of
there exists a corresponding value of 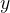 in the range
in the range  , such that the point
, such that the point 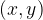 is incident to
is incident to 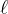 . That is, every line of
. That is, every line of  is incident to exactly
is incident to exactly  points of
points of 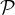 , and thus
, and thus
And that is the end of the construction! In the next post of the series, I plan to start surveying planar configurations of other types of curves.


Pingback: Incidences: Lower Bounds (part 3) | Some Plane Truths
The constant 0.63 for Elekes’s construction is mentioned in Roel Apfelbaum’s thesis, section 1.1.
Thank you Frank. That was nice catch!
Pingback: Incidences: Lower Bounds (part 6) | Some Plane Truths
Excellent post! I am in the process of giving a crash course on geometric combinatorics in my data science seminar. This construction is definitely the way to go!
Thanks Alex!
The calculation in the paper of Pach and Tóth contains a numerical error. The correct lower bound given by the Erdős construction is three times larger, that is 1.27. We have more details in the footnote on page 3 of https://arxiv.org/pdf/1703.04767v2
Right! I read your paper and saw this footnote before. Your new incidence bound for flats is very nice, and I should also have a post in the lower bounds series about it. I’ve been neglecting this series for a while…
I just updated this post accordingly. Thank you for pointing this out.
Pingback: Configurations with many incidences and no triangles – Cosmin Pohoata
Pingback: Incidences: Open Problems (part 2) – Some Plane Truths